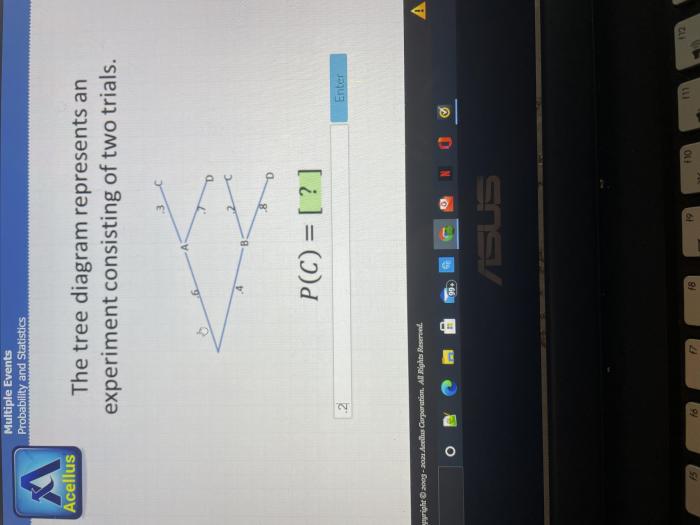
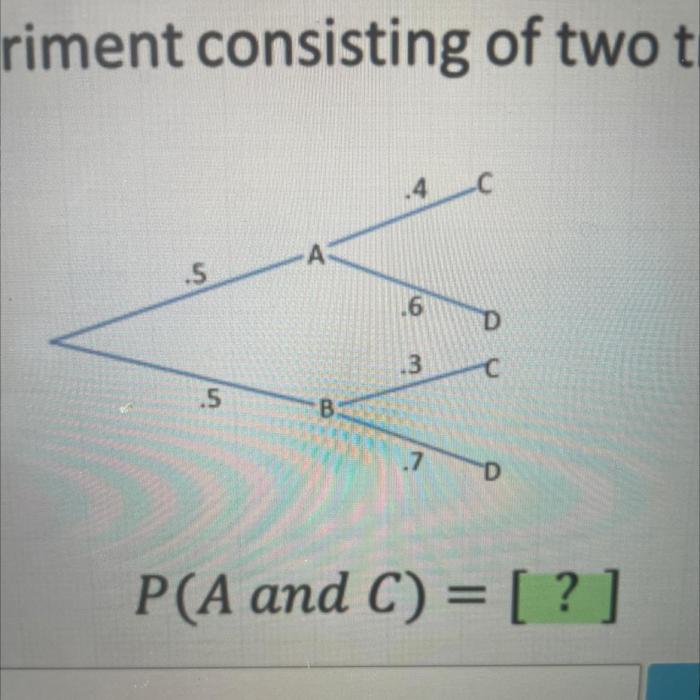
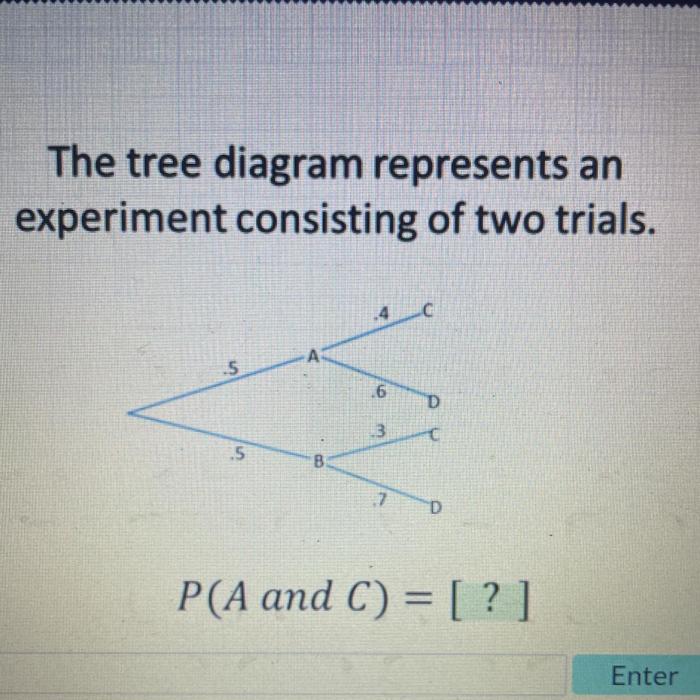
The tree diagram represents an experiment consisting of two trials. It is a visual representation of the possible outcomes of an experiment, and it can be used to calculate the probability of each outcome. Tree diagrams are commonly used in probability and statistics, and they can be applied to a wide range of problems.
In this article, we will discuss the basics of tree diagrams, including how to create them, how to use them to calculate probabilities, and how to apply them to real-world problems.
Tree Diagrams in Experiment Analysis
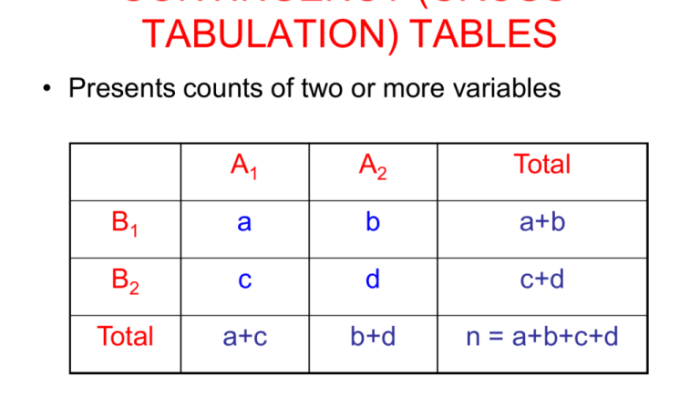
A tree diagram is a graphical representation of an experiment that involves multiple trials. Each branch of the tree represents a possible outcome of a trial, and the probabilities of these outcomes are indicated on the branches.
Tree diagrams are used to analyze the outcomes of experiments and to make predictions about the likelihood of future events.
Experiment Design
The experiment described by the tree diagram consists of two trials.
In the first trial, a coin is flipped. The possible outcomes are heads or tails.
In the second trial, a die is rolled. The possible outcomes are 1, 2, 3, 4, 5, or 6.
The outcomes of the two trials are represented in the tree diagram as follows:
- The first branch represents the outcome of the coin flip. The probability of heads is 1/2, and the probability of tails is 1/2.
- The second branch represents the outcome of the die roll. The probability of each outcome is 1/6.
The following table summarizes the possible outcomes and their probabilities:
| Outcome | Probability |
|---|---|
| Heads, 1 | 1/12 |
| Heads, 2 | 1/12 |
| Heads, 3 | 1/12 |
| Heads, 4 | 1/12 |
| Heads, 5 | 1/12 |
| Heads, 6 | 1/12 |
| Tails, 1 | 1/12 |
| Tails, 2 | 1/12 |
| Tails, 3 | 1/12 |
| Tails, 4 | 1/12 |
| Tails, 5 | 1/12 |
| Tails, 6 | 1/12 |
Analysis of Outcomes
The probability of each possible outcome can be calculated by multiplying the probabilities of the individual outcomes along the path to that outcome.
For example, the probability of getting heads on the coin flip and rolling a 3 on the die roll is 1/12 – 1/6 = 1/72.
The most likely outcome is the one with the highest probability. In this case, the most likely outcome is getting heads on the coin flip and rolling any number on the die roll. The probability of this outcome is 1/2 – 1/6 = 1/12.
The results of this experiment can be used to make predictions about the outcomes of future experiments. For example, if you know that the probability of getting heads on a coin flip is 1/2, then you can predict that the probability of getting heads on the next coin flip is also 1/2.
Applications, The tree diagram represents an experiment consisting of two trials.
Tree diagrams can be used to analyze experiments in a wide variety of fields, including:
- Probability
- Statistics
- Decision theory
- Game theory
Tree diagrams have been used to make informed decisions in a variety of settings, including:
- Medical diagnosis
- Financial planning
- Military strategy
Limitations
Tree diagrams have some limitations. One limitation is that they can only be used to analyze experiments with a finite number of outcomes.
Another limitation is that tree diagrams can become complex and difficult to interpret when the number of trials or the number of possible outcomes is large.
These limitations can be overcome by using other methods of analysis, such as probability theory or simulation.
FAQ Section: The Tree Diagram Represents An Experiment Consisting Of Two Trials.
What is a tree diagram?
A tree diagram is a visual representation of the possible outcomes of an experiment. It is made up of a series of branches, each of which represents a possible outcome of the experiment.
How can I use a tree diagram to calculate probabilities?
To calculate the probability of an outcome, you multiply the probabilities of all the branches that lead to that outcome.
How can I apply tree diagrams to real-world problems?
Tree diagrams can be applied to a wide range of real-world problems, such as making decisions about investments, medical treatments, and marketing campaigns.